

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Vector Spherical Harmonic
المؤلف:
Arfken, G.
المصدر:
"Vector Spherical Harmonics." §12.11 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press
الجزء والصفحة:
...
25-9-2019
2928
The spherical harmonics can be generalized to vector spherical harmonics by looking for a scalar function 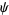 and a constant vector
and a constant vector 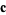 such that
such that
 |
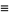 |
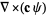 |
(1) |
 |
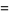 |
 |
(2) |
 |
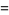 |
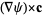 |
(3) |
 |
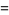 |
 |
(4) |
so
 |
(5) |
Now interchange the order of differentiation and use the fact that multiplicative constants can be moving inside and outside the derivatives to obtain
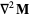 |
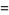 |
 |
(6) |
 |
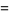 |
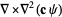 |
(7) |
 |
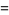 |
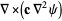 |
(8) |
and
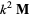 |
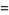 |
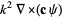 |
(9) |
 |
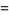 |
 |
(10) |
Putting these together gives
![del ^2M+k^2M=del x[c(del ^2psi+k^2psi)],](http://mathworld.wolfram.com/images/equations/VectorSphericalHarmonic/NumberedEquation2.gif) |
(11) |
so 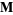 satisfies the vector Helmholtz differential equation if
satisfies the vector Helmholtz differential equation if 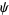 satisfies the scalar Helmholtz differential equation
satisfies the scalar Helmholtz differential equation
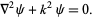 |
(12) |
Construct another vector function
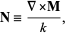 |
(13) |
which also satisfies the vector Helmholtz differential equation since
 |
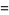 |
 |
(14) |
 |
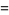 |
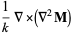 |
(15) |
 |
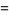 |
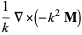 |
(16) |
 |
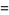 |
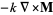 |
(17) |
 |
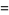 |
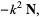 |
(18) |
which gives
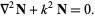 |
(19) |
We have the additional identity
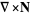 |
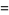 |
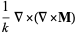 |
(20) |
 |
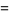 |
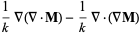 |
(21) |
 |
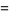 |
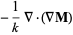 |
(22) |
 |
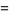 |
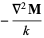 |
(23) |
 |
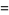 |
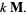 |
(24) |
In this formalism, 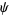 is called the generating function and
is called the generating function and  is called the pilot vector. The choice of generating function is determined by the symmetry of the scalar equation, i.e., it is chosen to solve the desired scalar differential equation. If
is called the pilot vector. The choice of generating function is determined by the symmetry of the scalar equation, i.e., it is chosen to solve the desired scalar differential equation. If 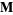 is taken as
is taken as
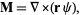 |
(25) |
where  is the radius vector, then
is the radius vector, then 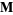 is a solution to the vector wave equation in spherical coordinates. If we want vector solutions which are tangential to the radius vector,
is a solution to the vector wave equation in spherical coordinates. If we want vector solutions which are tangential to the radius vector,
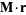 |
 |
 |
(26) |
 |
 |
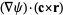 |
(27) |
 |
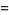 |
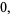 |
(28) |
so
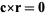 |
(29) |
and we may take
 |
(30) |
(Arfken 1985, pp. 707-711; Bohren and Huffman 1983, p. 88).
A number of conventions are in use. Hill (1954) defines
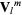 |
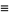 |
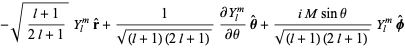 |
(31) |
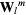 |
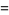 |
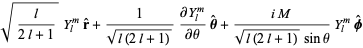 |
(32) |
 |
 |
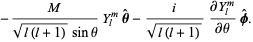 |
(33) |
Morse and Feshbach (1953) define vector harmonics called 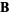 ,
, 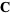 , and
, and 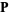 using rather complicated expressions.
using rather complicated expressions.
REFERENCES:
Arfken, G. "Vector Spherical Harmonics." §12.11 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 707-711, 1985.
Blatt, J. M. and Weisskopf, V. "Vector Spherical Harmonics." Appendix B, §1 in Theoretical Nuclear Physics. New York: Wiley, pp. 796-799, 1952.
Bohren, C. F. and Huffman, D. R. Absorption and Scattering of Light by Small Particles. New York: Wiley, 1983.
Hill, E. H. "The Theory of Vector Spherical Harmonics." Amer. J. Phys. 22, 211-214, 1954.
Jackson, J. D. Classical Electrodynamics, 2nd ed. New York: Wiley, pp. 744-755, 1975.
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part II. New York: McGraw-Hill, pp. 1898-1901, 1953.