The Delannoy numbers 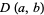 are the number of lattice paths from  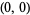to  in which only east (1, 0), north (0, 1), and northeast (1, 1) steps are allowed (i.e., 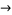, , and 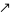). They are given by the recurrence relation

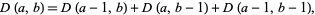(1)

with 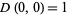. The are also given by the sums

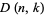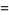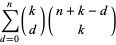			(2)
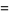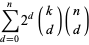			(3)
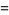			(4)

where 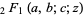 is a hypergeometric function.

A table for values for the Delannoy numbers is given by

(5)

(OEIS A008288) for 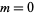, 1, ... increasing from left to right and , 1, ... increasing from top to bottom.

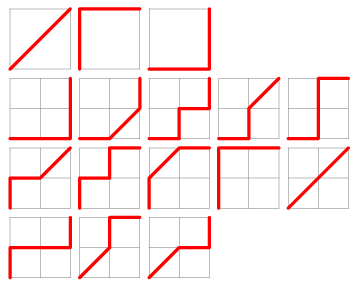
They have the generating function

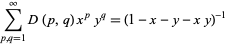(6)

(Comtet 1974, p. 81).

Taking 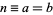 gives the central Delannoy numbers , which are the number of "king walks" from the 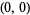 corner of an  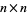square to the upper right corner 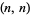. These are given by

(7)

where 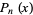 is a Legendre polynomial (Moser 1955; Comtet 1974, p. 81; Vardi 1991). Another expression is

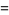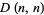			(8)
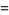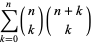			(9)
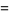			(10)

where 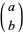 is a binomial coefficient and  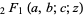is a hypergeometric function. These numbers have a surprising connection with the Cantor set (E. W. Weisstein, Apr. 9, 2006).

They also satisfy the recurrence equation

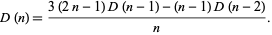(11)

They have generating function

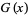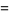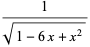			(12)
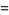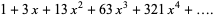			(13)

The values of 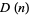 for 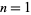, 2, ... are 3, 13, 63, 321, 1683, 8989, 48639, ... (OEIS A001850). The numbers of decimal digits in  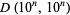for 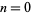, 1, ... are 1, 7, 76, 764, 7654, 76553, 765549, 7655510, ... (OEIS A114470), where the digits approach those of  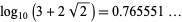(OEIS A114491).

The first few prime Delannoy numbers are 3, 13, 265729, ... (OEIS A092830), corresponding to indices 1, 2, 8, ..., with no others for 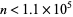 (Weisstein, Mar. 8, 2004).

The Schröder numbers bear the same relation to the Delannoy numbers as the Catalan numbers do to the binomial coefficients.

Amazingly, taking the Cholesky decomposition of the square array of , transposing, and multiplying it by the diagonal matrix 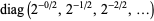 gives the square matrix (i.e., lower triangular) version of Pascal's triangle (G. Helms, pers. comm., Aug. 29, 2005).

Beautiful fractal patterns can be obtained by plotting  (mod 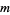) (E. Pegg, Jr., pers. comm., Aug. 29, 2005). In particular, the  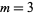case corresponds to a pattern resembling the Sierpiński carpet.

REFERENCES:

Banderier, C. and Schwer, S. "Why Delannoy Numbers?" To appear in J. Stat. Planning Inference. http://www-lipn.univ-paris13.fr/~banderier/Papers/delannoy2004.ps.

Comtet, L. Advanced Combinatorics: The Art of Finite and Infinite Expansions, rev. enl. ed. Dordrecht, Netherlands: Reidel, pp. 80-81, 1974.

Dickau, R. M. "Delannoy and Motzkin Numbers." http://www.prairienet.org/~pops/delannoy.html.

Goodman, E. and Narayana, T. V. "Lattice Paths with Diagonal Steps." Canad. Math. Bull. 12, 847-855, 1969.

Moser, L. "King Paths on a Chessboard." Math. Gaz. 39, 54, 1955.

Moser, L. and Zayachkowski, H. S. "Lattice Paths with Diagonal Steps." Scripta Math. 26, 223-229, 1963.

Sloane, N. J. A. Sequences A001850/M2942, A008288 , A092830, A114470, and A114491 in "The On-Line Encyclopedia of Integer Sequences."

Stocks, D. R. Jr. "Lattice Paths in 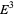 with Diagonal Steps." Canad. Math. Bull. 10, 653-658, 1967.

Vardi, I. Computational Recreations in Mathematica. Reading, MA: Addison-Wesley, 1991.